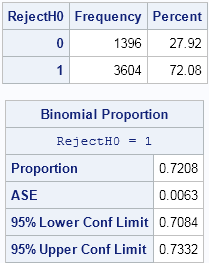
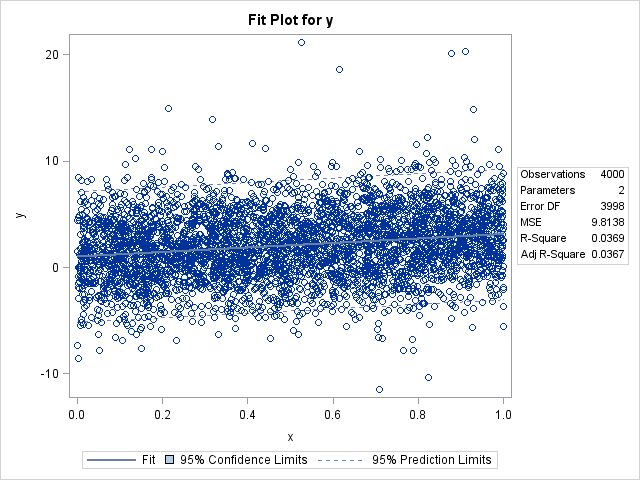
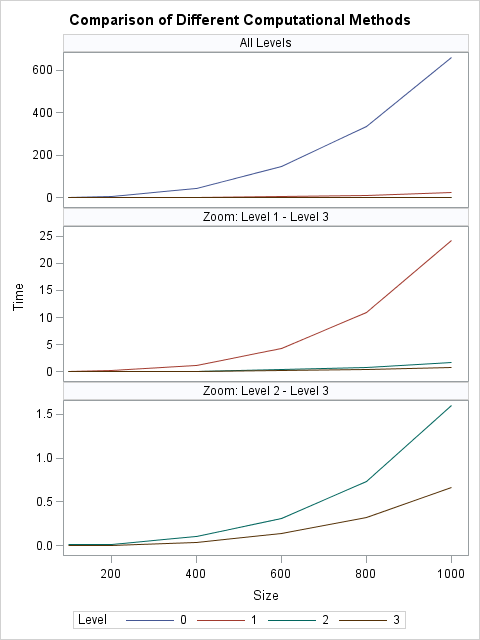
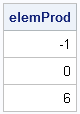
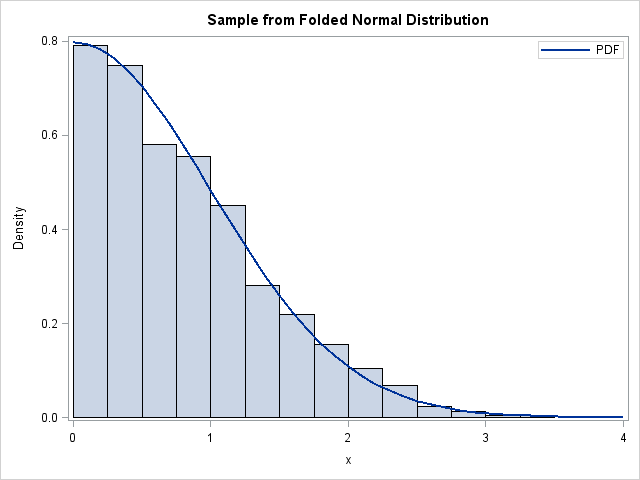
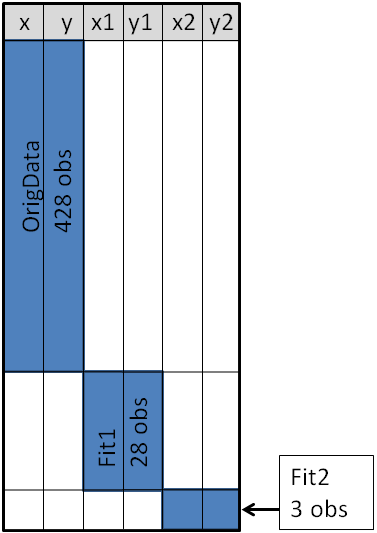
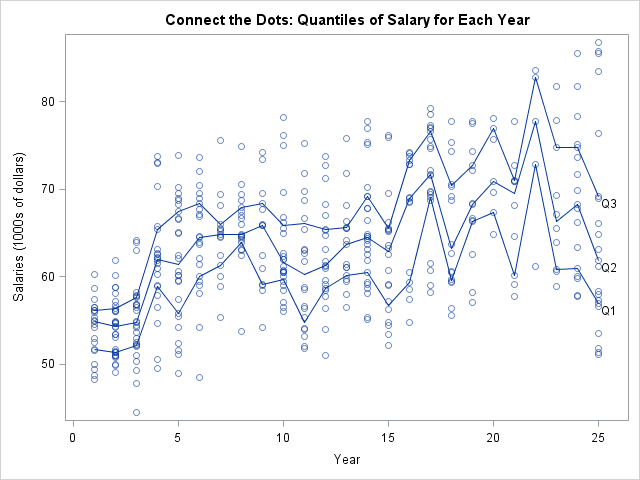
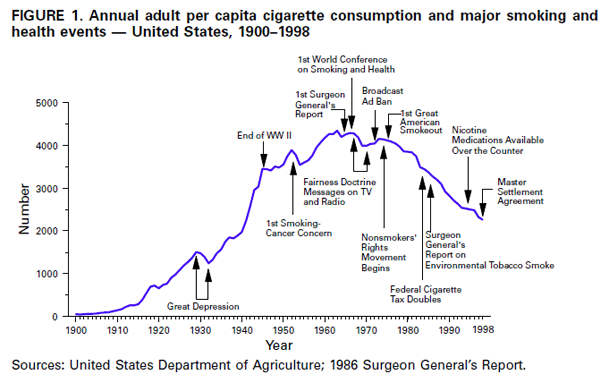
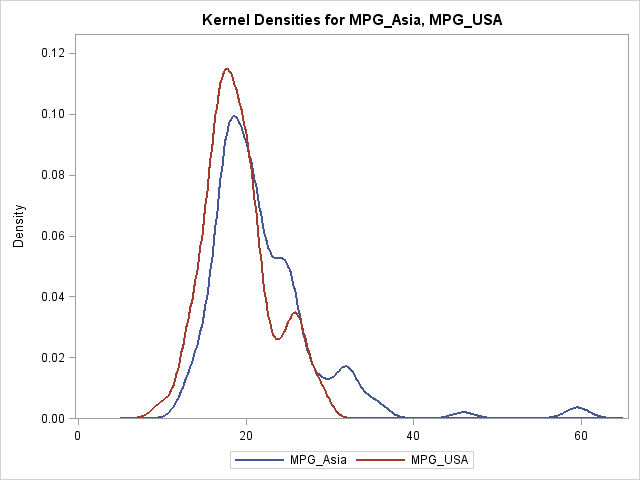
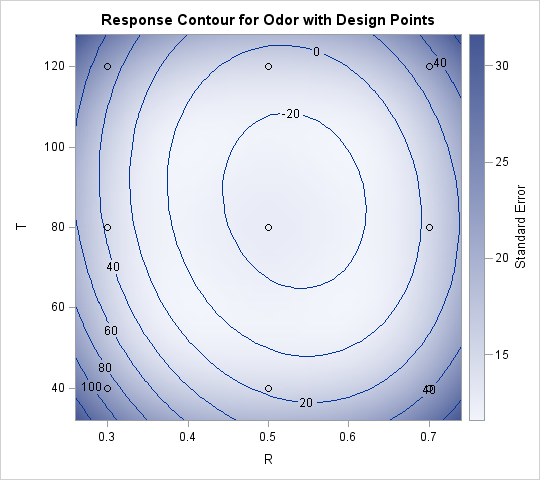
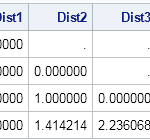
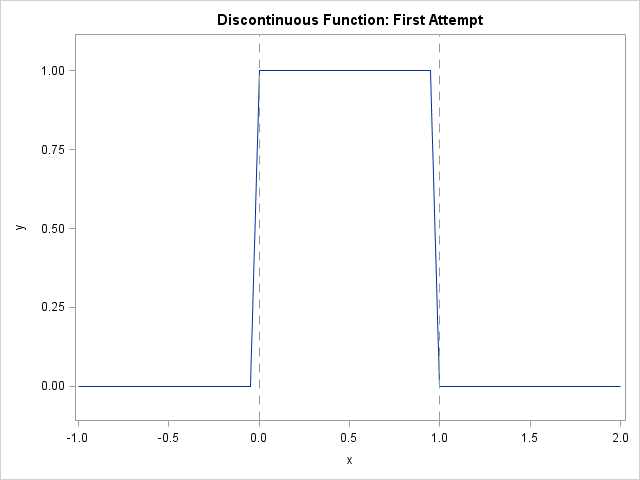
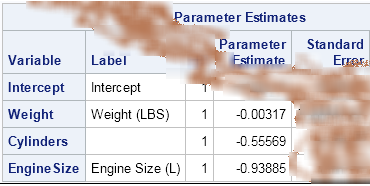
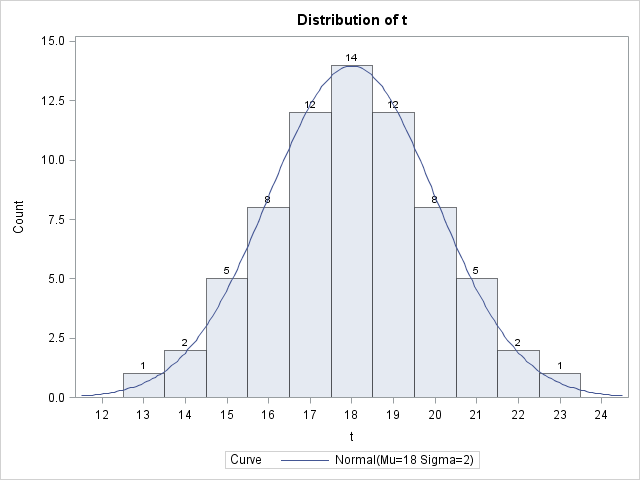
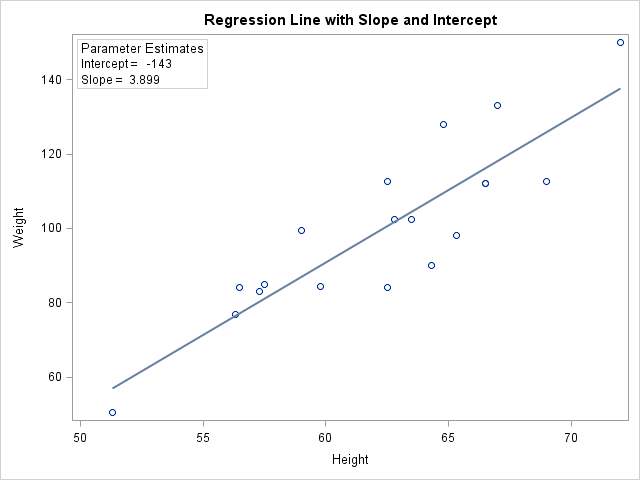
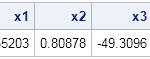A SAS user told me that he computed a vector of values in the SAS/IML language and wanted to use those values on a statement in a SAS procedure. The particular application involved wanting to use the values on the ESTIMATE and CONTRAST statements in a SAS regression procedure, but