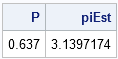
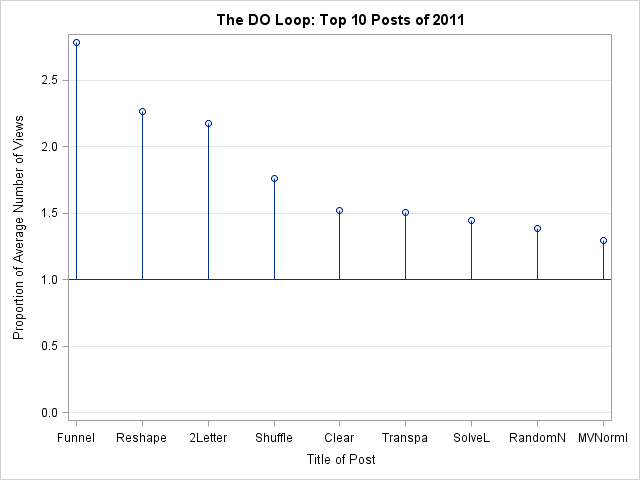
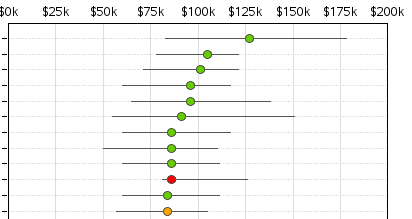
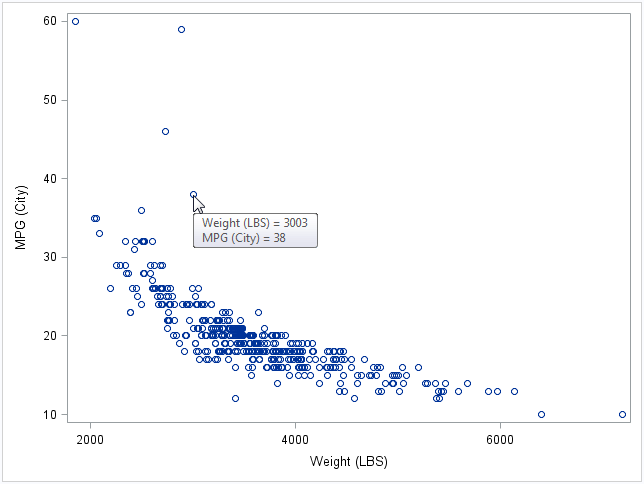
If you live in the US, do you remember the Dunkin Donuts commercial from about 30 years ago. It opens with a devoted donut maker climbing out of bed, saying "It's time to make the donuts." (Donut makers have to get up early so that you and I can have
Following up on the theme of graphs commonly used in many applications, here is another one that displays a horizontal bar chart with multiple data columns aligned with the bars. Recently at WUSS, a user stopped by asking how to create just such a graph. While this user wanted something more complex
Buffon's needle experiment for estimating π is a classical example of using an experiment (or a simulation) to estimate a probability. This example is presented in many books on statistical simulation and is famous enough that Brian Ripley in his book Stochastic Simulation states that the problem is "well known
In the immortal words of Britney Spears: Oops! I did it again. At least, I'm afraid that I did. I think I might have helped a SAS student with a homework assignment, or perhaps provided an answer in preparation for a SAS certification exam. Or maybe it was a legitimate
Local governments are not immune to the rising demand for more accountability in government run services and programs, and the expenditure of public funds. Elected officials and citizens alike want to know what these programs accomplish for the public investment made. To date, the response to these questions and demands has been
Let us ring in the new year with something simple and useful. A recent question by a user over the holidays motivated this article on what is likely a commonly used graph. We want to compare the preformance of two categories along with a third measure. This could be something like "How
Hello, 2012! It's a New Year and I'm flushed with ideas for new blog articles. (You can also read about The DO Loop's most popular posts of 2011.) The fundamental purpose of my blog is to present tips and techniques for writing efficient statistical programs in SAS. I pledge to
Today, every organization is running on too few resources - getting it done with fewer people, a reduced budget and less time. Grid computing enables SAS to automatically use a centrally managed grid infrastructure to achieve workload balancing, high availability and parallel processing. Do what once took days in minutes
At the beginning of 2011, I made four New Year's resolutions for my blog. As the year draws to a close, it's time to see how I did: Resolution: 100 blog posts in 2011: Completed. I blew by this goal by posting 165 articles. I recently compiled a list of
It’s a time for excitement, laughter, getting together with friends and family, and best of all cookie exchanges! It’s kind of sad that in a few days it will all be over. Does it have to end? Can’t another holiday or event bring about the same feelings? How about SAS
As you probably remember, this innovation series is really Brainstorming 2.0. I've taken the Post-It Notes that SAS users put on the Innovation Wall and reposted them here to help spark new ideas for using SAS. I'm also adding posts here and there when I have an interview with a customer
Lots of the visitors to this blog arrive here by way of Google search (welcome!). Thanks to search engines and a few well placed keywords, the same older posts (let's call them "timeless topics") seem to attract the most traffic from year to year. I hope that the searchers find
When I give presentations on using the SG procedures, I try to describe how you can take simple plots and layer them to create more complex graphs. I also emphasize how you must consider the output of each plot type so that, as you overlay them, you do not obscure
2011 saw incredible change in the states as more than half of the governorships changed hands. As expected, that caused significant turnover at the State CIO level, as well. So while executive and technology policies changed in many ways, the dismal budget situation reinforced the need to allocate tax pay
In Simple maps can go a long way, we discussed some techniques to create simple outline maps from map datasets in the MAPS library using GTL. Now, let us take this a step further to do something more useful with this feature. For some graphs, the map information is an essential part of the
On the heels of the release of the popular SAS macro variable viewer from last month, I'm providing another custom task that I hope will prove just as useful. This one is a SAS options viewer, similar in concept to the OPTIONS window in SAS display manager. You can download
From the largest corporation to a small startup, data overload can be a crippling problem. Just ask this big guy ... Now you know. Happy holidays!
A few colleagues and I were exchanging short snippets of SAS code that create Christmas trees and other holiday items by using the SAS DATA step to arrange ASCII characters. For example, the following DATA step (contributed by Udo Sglavo) creates a Christmas tree with ornaments and lights: data _null_;
A new book from SAS Press, "Statistical Graphics Procedures by Example" co-authored by Dan Heath and I has now been published (phew!). For both Dan and I, this was our first foray into writing a book, so it was highly educational to say the least. The key idea behind the presentation
After my November blog post about achievement gaps between affluent and poor students, Newt Gingrich cast some renewed energy on the topic with his inflammatory comments about poor children at a Dec 1st GOP debate. In case you missed his remarks, here is the quote that sent the media into
My daughter's math lessons this year have included the concept of negative numbers (that is, numbers that are less than zero, not numbers that have a bad attitude). She has used number lines such as this one to help her while she completes her homework: Notice that in this number
Since this is a blog about statistical programming and analysis, I am always looking for data to analyze. As 2011 ends, I look back on the 165 blog entries that I published since 01JAN2011. This article presents the 10 most popular posts, as determined by the number of people who
On his SAS and R blog, Ken K. recently posted an example of a visualization technique called "small multiples". In this exercise, Ken shows the programming technique for replicating a particular series of pie charts in R as well as in SAS. It's a useful exercise to learn from, but
Charlie Huang recently posted an article on a new way to draw maps using SGPlot procedure. The basic idea is simple, just use the SCATTER statement to plot the (x, y) points from the data sets in the MAPS library. The GROUP option can be used to color the markers for each
Since joining SAS, I no longer run screaming from social media, blogs, etc. In fact, I embrace exploring different mediums to highlight how SAS is assisting today’s law enforcement. To that end, and in recognition that sometimes less is more, I give you this short intro. SAS continues to push
The SAS Output Delivery System (ODS) enables you to manage and customize tables (and graphics!) that are created by SAS procedures. I like to use the ODS SELECT statement to display only part of the output of a SAS procedure. For example, the UNIVARIATE procedure produces five tables by default,
The holiday season has just begun here in Cary, North Carolina. My favorite holiday is Christmas, but your's may be another. I'll try to outline some of the ones that are celebrated here and for some of my other global readers - I may miss an important holiday when I try
Some SAS products such as SAS/IML Studio (which is included FREE as part of SAS/IML software) have interactive graphics. This makes it easy to interrogate a graph to determine values of "hidden" variables that might not appear in the graph. For example, in a scatter plot in SAS/IML Studio, you
Calendar Heatmaps are an interesting alternative view of time-series data. The measured value is displayed as color mapped cells in a calendar. Calendar Heatmaps can be easily created with SAS 9.3 using just the HEATMAPPARM, SERIESPLOT and BLOCKPLOT statements in GTL and some simple data manipulation. The example below shows
In one minute (and 10 seconds, but are you measuring that?) you can add a custom measure (also known as calculated members) to your OLAP cube. Watch this video to create a simple calculation multiplying units * price. Remember that this mechanism is not just used for multiplications, you can
