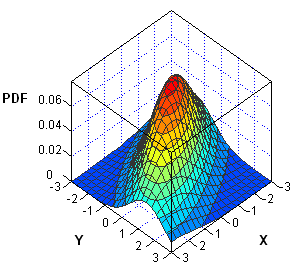
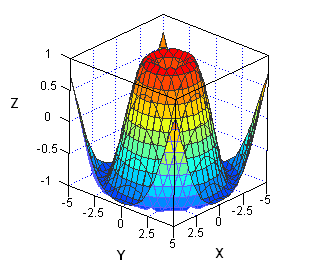
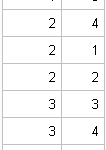
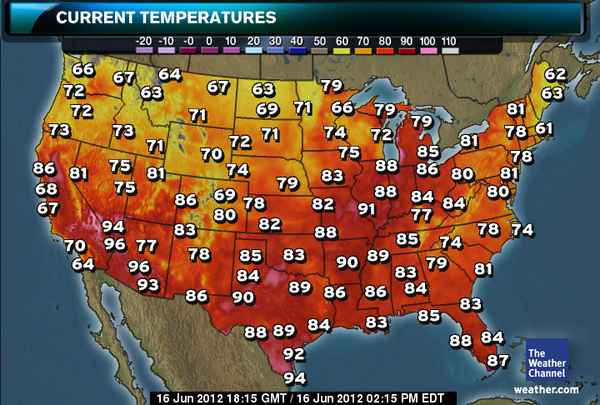
About cookies on this site
This site uses cookies and related technologies for site operation, analytics and third-party advertising purposes, as described in our SAS Privacy Statement. You may consent to our use of these technologies, reject non-essential technologies or further manage your preferences. To opt out of SAS making information relating to cookies and similar technologies available to third parties for advertising purposes, select "Required only." To exercise other rights you may have related to cookies, select "Manage cookies."