The DO Loop
Statistical programming in SAS with an emphasis on SAS/IML programs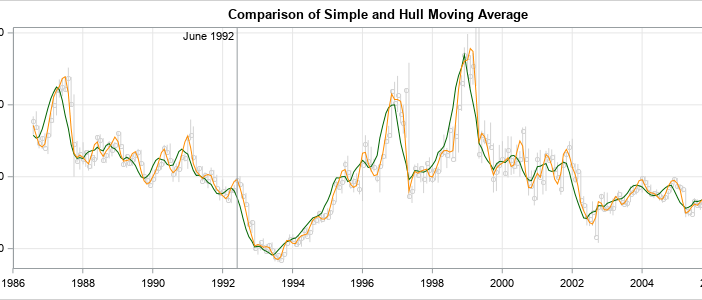
A moving average is a statistical technique that is used to smooth a time series. My colleague, Cindy Wang, wrote an article about the Hull moving average (HMA), which is a time series smoother that is sometimes used as a technical indicator by stock market traders. Cindy showed how to
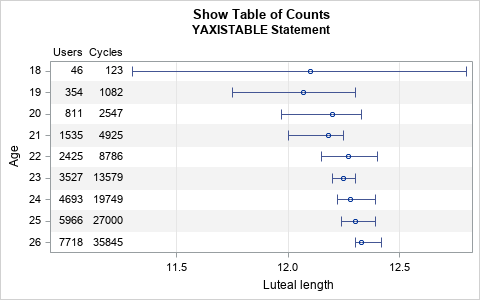
I often use axis tables in PROC SGPLOT in SAS to add a table of text to a graph so that the table values are aligned with the data. But axis tables are not the only way to display tabular data in a graph. You can also use the TEXT
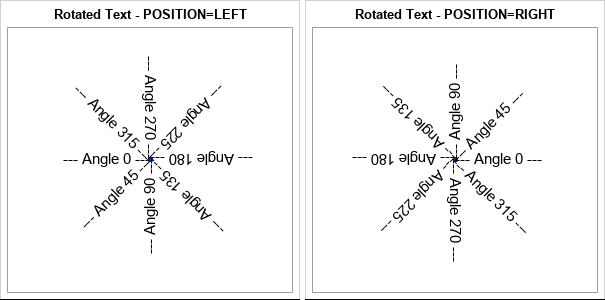
The TEXT statement in PROC SGPLOT supports the ROTATE= option to rotate the specified text. It is worth knowing how the ROTATE= option interacts with the POSITION= option, which determines the anchor point at which the text is positioned. Briefly, the text is positioned FIRST, then the rotation occurs. The
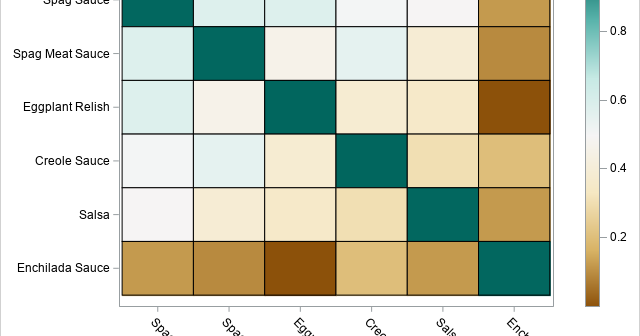
When you order an item online, the website often recommends other items based on your purchase. In fact, these kinds of "recommendation engines" contributed to the early success of companies like Amazon and Netflix. SAS uses a recommender engine to suggest articles on the SAS Support Communities. Although recommender engines
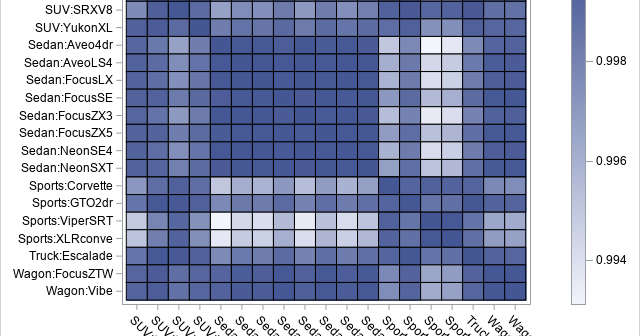
An important application of the dot product (inner product) of two vectors is to determine the angle between the vectors. If u and v are two vectors, then cos(θ) = (u ⋅ v) / (|u| |v|) You could apply the inverse cosine function if you wanted to find θ in
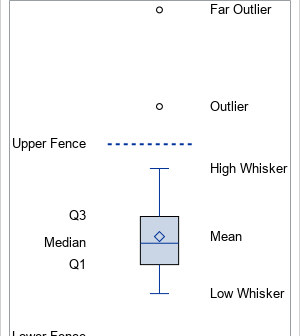
A SAS programmer asked an intriguing question on the SAS Support Communities: Can you use SAS to create a graph that shows how the elements in a box-and-whiskers plot relate to the data? The SAS documentation has several examples that explain how to read a box plot. One of the
