The DO Loop
Statistical programming in SAS with an emphasis on SAS/IML programs
SAS software contains a lot of features, and each release adds more.To make sure that you do not miss new features that appear in the SAS/IML language, the word cloud on the right sidebar of my blog contains numbers that relate to SAS or SAS/IML releases. For example, you can
Have you ever noticed that some SAS/IML programmers use the CALL statement to call a subroutine, whereas others use the RUN statement? Have you ever wondered why the SAS/IML language has two statements that do the same thing? It turns out that the CALL statement and the RUN statement do
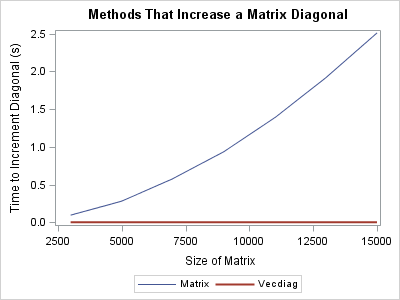
I was recently asked about how to use the SAS/IML language to efficiently add a constant to every element of a matrix diagonal. Mathematically, the task is to form the matrix sum A + kI, where A is an n x n matrix, k is a scalar value, and I is the

It has been three months since the introduction of the SAS/IML File Exchange, so I thought I'd give a short update on recent submissions and activity. (Note: The File Exchange was merged into the SAS Community Library in 2022.) Users have submitted eight new articles. The application areas include experimental
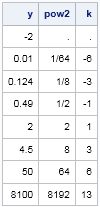
The other day I was doing some computations that caused me to wonder, "What is the smallest power of 2 that is greater than a given number?" The mathematics is straightforward. Given a number n, find the least value of k such that 2k ≥ n or, equivalently, k ≥
SAS/IML 13.1 includes a handy function for programmers who write a lot of modules. The PARENTNAME function obtains the name of the symbol that was passed in as a parameter to a user-defined module. How is this useful? Well, suppose that you want to create a SAS/IML module that prints
