Rick Wicklin, PhD, is a distinguished researcher in computational statistics at SAS and is a principal developer of SAS/IML software. His areas of expertise include computational statistics, simulation, statistical graphics, and modern methods in statistical data analysis. Rick is author of the books Statistical Programming with SAS/IML Software and Simulating Data with SAS.
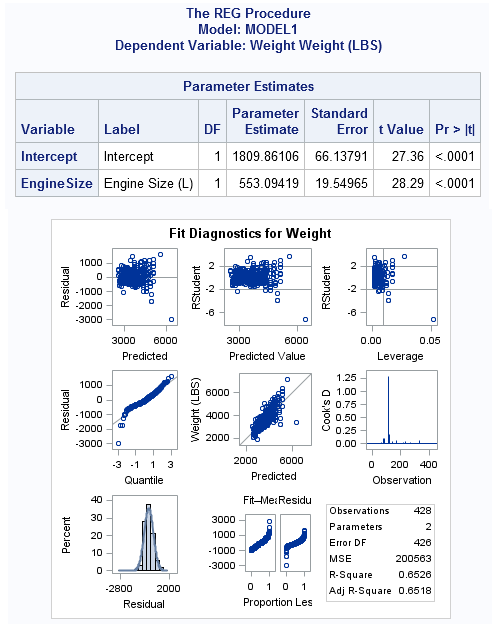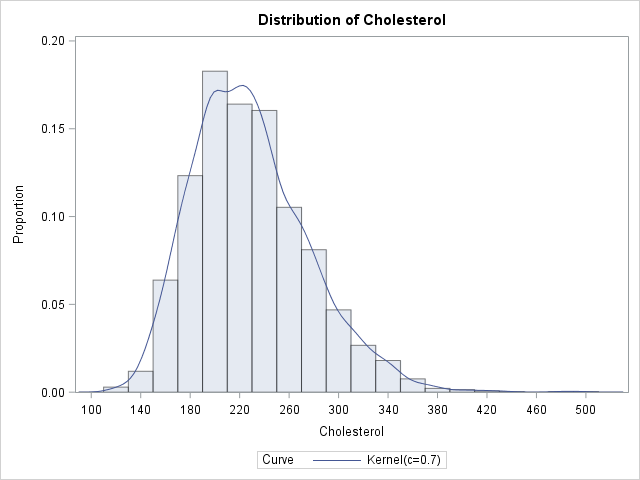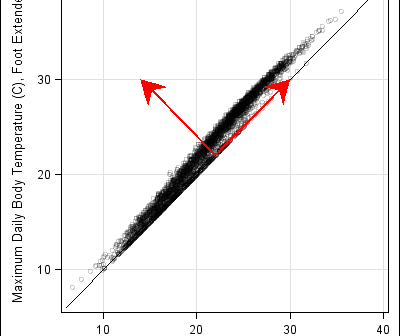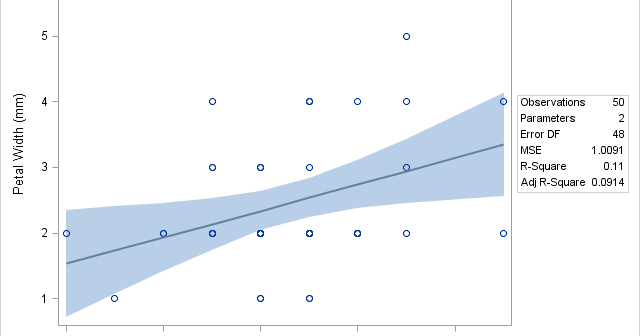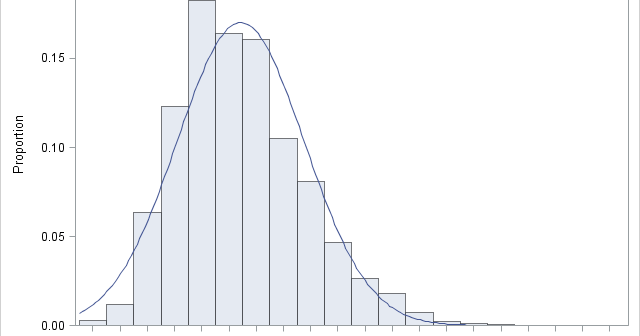
Welcome, SAS 9.3! I've already blogged about some interface and graphical changes that everyone should know about. Now I'll put on my statistical hat and mention a few 9.3 features that excite me, personally, as a data analyst and a statistical programmer: As a statistician, I am keen to try
Here are a few new interface and graphics changes that every SAS programmer should know about SAS 9.3: HTML is now the default output destination when you run the SAS windowing environment. This means that tables and graphs appear in an HTML document instead of the classic LISTING destination. Of
As I was reviewing notes for my course "Data Simulation for Evaluating Statistical Methods in SAS," I realized that I haven't blogged about simulating categorical data in SAS. This article corrects that oversight. An Easy Way and a Harder Way SAS software makes it easy to sample from discrete "named"
Arnold Loewy, professor of criminal law at Texas Tech University, wrote an editorial about the Casey Anthony case that has statistical undertones. Prof. Loewy discusses the fact that there are two kinds of errors that can occur in a court trial: an innocent person can be sent to jail or
"Always clean up after yourself." My mother taught me this, and I apply it to SAS programming as regularly as I apply it at home. For SAS programming, I reinterpret Mom's saying as the following rule: Always delete temporary files and data sets when you are finished using them. How
One of the joys of statistics is that you can often use different methods to estimate the same quantity. Last week I described how to compute a parametric density estimate for univariate data, and use the parameters estimates to compute the area under the probability density function (PDF). This article
If you create a scatter plot of highly correlated data, you will see little more than a thin cloud of points. Small-scale relationships in the data might be masked by the correlation. For example, Luke Miller recently posted a scatter plot that compares the body temperature of snails when they
In a previous article, I discussed random jittering as a technique to reduce overplotting in scatter plots. The example used data that are rounded to the nearest unit, although the idea applies equally well to ordinal data in general. The act of jittering (adding random noise to data) is a
Jittering. To a statistician, it is more than what happens when you drink too much coffee. Jittering is the act of adding random noise to data in order to prevent overplotting in statistical graphs. Overplotting can occur when a continuous measurement is rounded to some convenient unit. This has the
The area under a density estimate curve gives information about the probability that an event occurs. The simplest density estimate is a histogram, and last week I described a few ways to compute empirical estimates of probabilities from histograms and from the data themselves, including how to construct the empirical
